ARCHIVED CONTENT: In December 2020, the CZO program was succeeded by the Critical Zone Collaborative Network (CZ Net)
×
Le et al., 2018
Stochastic Lattice-Based Modelling of Malaria Dynamics
Le, P.V.V., Kumar, P., and Ruiz, M.O. (2018)
Malaria Journal
-
IML, GRAD STUDENT
-
IML, INVESTIGATOR
Abstract
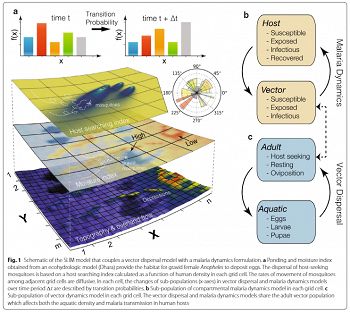
Schematic of the SLIM model.
Background: The transmission of malaria is highly variable and depends on a range of climatic and anthropogenic factors. In addition, the dispersal of Anopheles mosquitoes is a key determinant that affects the persistence and dynamics of malaria. Simple, lumped-population models of malaria prevalence have been insufficient for predicting the complex responses of malaria to environmental changes.
Methods and results: A stochastic lattice-based model that couples a mosquito dispersal and a susceptible-exposed-infected-recovered epidemics model was developed for predicting the dynamics of malaria in heterogeneous environments. The Itˆo approximation of stochastic integrals with respect to Brownian motion was used to derive a model of stochastic differential equations. The results show that stochastic equations that capture uncertainties in the life cycle of mosquitoes and interactions among vectors, parasites, and hosts provide a mechanism for the disruptions of malaria. Finally, model simulations for a case study in the rural area of Kilifi county, Kenya are presented.
Conclusions: A stochastic lattice-based integrated malaria model has been developed. The applicability of the model for capturing the climate-driven hydrologic factors and demographic variability on malaria transmission has been demonstrated.
Citation
Le, P.V.V., Kumar, P., and Ruiz, M.O. (2018): Stochastic Lattice-Based Modelling of Malaria Dynamics. Malaria Journal. DOI: doi.org/10.1186/s12936-018-2397-z
 This Paper/Book acknowledges NSF CZO grant support.
This Paper/Book acknowledges NSF CZO grant support.
Explore Further