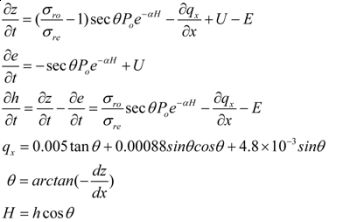One of the objectives of the Shale Hills Critical Zone Observatory is to understand how landscape morphology arises from feedbacks among hydrological, hillslope, and fluvial processes.  Towards this end we are constructing a next-generation 3D landscape evolution model (PIHMSed) that couples the processes of bedrock uplift, weathering, and regolith transport.  The model is based upon PIHM, the Penn State Integrated Hydrologic Model, but also includes regolith and soil transport and evolving bedrock and soil-air interfaces.  The relevant conservation equations are discretized on a TIN (see figure) using the semi-discrete finite volume approach.
Among the processes causes regolith flux on hillslopes, tree throw is considered particularly important at Shale Hills.  We use the sine function suggested by Gabet, Reichman et al. (2003) to describe lateral regolith flux caused by tree throw: q_tr = K sin α, where K is a coefficient that depends upon the frequency of tree-fall per unit area, width of the root plate, and pit depth, and taken at Shale Hills to be 4.8*10-3 m2/year. Streams are modeled as regions of the triangular mesh where the overland flow exceeds a user-supplied threshold.

Definition sketch of hillslope
Consider a hillslope where the variables are defined as z = ground surface elevation (m), e = bedrock interface elevation (m), h = regolith thickness in vertical (m), U = rock uplift rate (positive upwards) (m yr-1), E = the net surface erosion rate (negative for deposition) (m yr-1) on the surface by overland flow, and qx = the lateral volumetric regolith flux rate (positive in the x-dir) (m3 m-1 yr-1) entering the sides of the control volume.  We want to predict the evolution of the ground elevation and regolith thickness as functions of location (x) (units of meters) and time (t) (measured in years to be consistent with standard practice).   Let the regolith bulk density be σ_re and the rock bulk density be σ_ro.
Then from conservation of mass:

Conversion of mass equation
Initial conditions for z, h, and e must be specified.  Typical boundary conditions are no lateral sediment flux at the drainage divide and a fixed ground elevation in the valley as set, for example, by an under-capacity stream that can keep up with the bedrock uplift rate.